The State of Matter
{Keith Dixon-Roche © 17/04/18}In this context, 'matter' is the term used to describe a collection of pure elements in their natural state at any temperature and/or pressure. Same-element matter refers to that in which all the atoms have the same atomic number. All these calculations refer to same-element matter.⁽¹⁾
The state of matter refers to its condition; viscous or gaseous; the only two conditions that exist for all matter.
The state of matter is entirely dependent upon its inter-atomic forces, from which we can predict all of its properties accurately using mathematics. In other words, we should no longer need to rely on documented data, which usually varies (sometimes significantly); most sources simply copy data from other sources - that may or may not be reliable - without verification.
But before we begin;
1) It has become clear that very little we see, hear and read concerning the state of matter is either accurate or true.
2) The term 'fluid' is a misnomer as it unites two disparate conditions; viscous and gaseous. All liquids are in a viscous state, gases are not.
3) There is no such property as the 'melting point' of matter. All matter will flow when it can no longer hold its shape under the influence of gravity. In other words, the 'melting point' of any matter will differ on the earth and the moon.
4) Hydrogen, either as a single proton or as a proton-electron pair, cannot exist in viscous form due to their positive [proton] electrical charges, which can only repel. Only as deuterium or tritium (containing one or two neutrons respectively) can this occur⁽³⁾.
5) Refer to our elemental data web-page for documented and calculated (CalQ) values of elemental densities and gas-transition temperatures.
The highest energy (e.g. temperature {Ṯ} or electro-magnetic {EME}) in any atom occurs in the proton-electron pairs whose electrons are orbiting in shell-1. All the following calculations are therefore based upon the performance of the proton-electron energies in these proton-electron pairs, whose electrons are orbiting in shell-1, and designated in the calculations with the sub-script '1'; e.g. PE₁, KE₁, etc.
Introduction
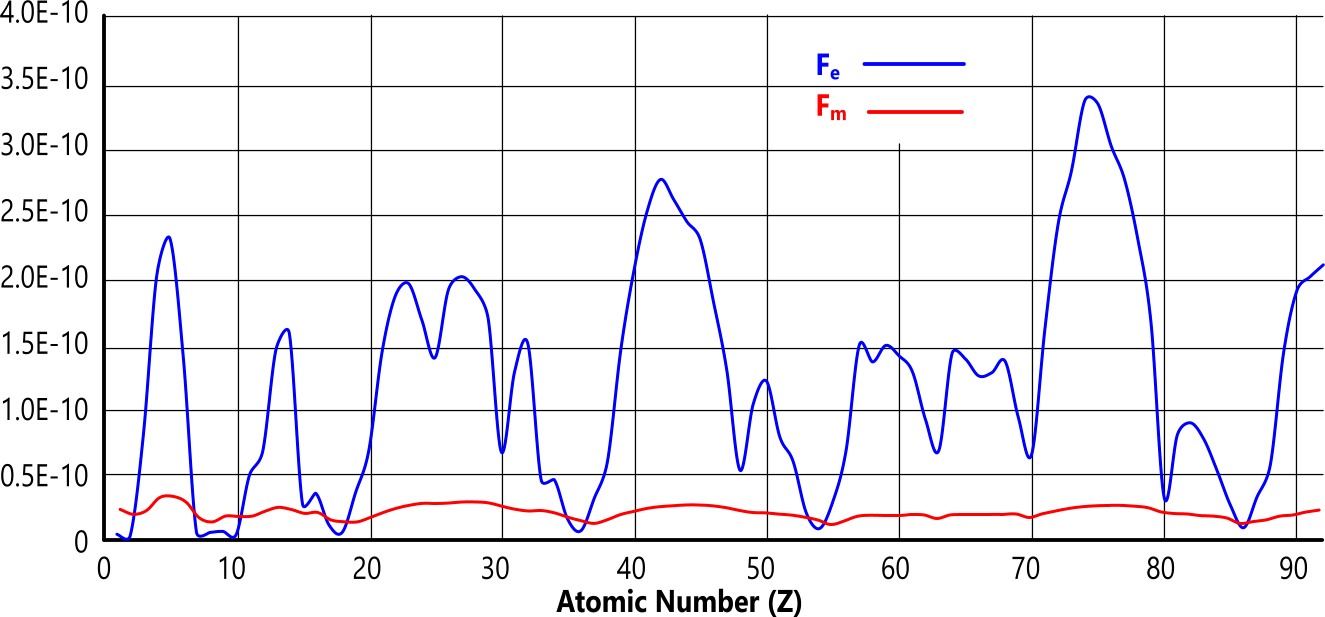
This work was initiated due to the discovery that a direct relationship appears to exist between the density of matter and its gas-transition temperature, as can be seen in Fig 1.
Its purpose is to answer the following question:
1) Can the Newton-Coulomb atomic model be used to predict the properties of elemental matter using mathematics?
Temperatures and Densities
The temperature we measure in matter is the EME generated by the proton-electron pairs (2-off#) whose electrons are orbiting in shell-1; the atom's innermost shell.
# All hydrogen atoms have only one orbiting electron in shell-1; all other atoms have two.
Density is the sum of atomic masses contained in a unit volume at a measured temperature. It varies with temperature in viscous matter but only slightly, due to the dominant constant magnetic forces. Same-density matter in gaseous form induces internal pressure that varies linearly with temperature.
Ṯ in these calculations is the temperature we measure in matter, which applies to that of the proton-electron pairs whose electrons are orbiting in Shell-1 of its constituent atoms (Ṯ₁).
The gas transition temperatures and densities used in the following calculations and plots are listed in the Table to the right of this page:
Fₑ refers to the electrical repulsion inter-atomic force {N) at a temperature of 300K,
Fₘ refers to the magnetic field attraction inter-atomic force {N) at a temperature of 300K,
ρ refers to the documented viscous density of the elements according to various sources,
Ṯg refers to the calculated (CalQ) gas-transition temperature of the elements.
It is important to understand that 'boiling point' is the temperature at which matter vapourises. It remains in a viscous state; globules suspended in atmospheric gases. Vapourisation requires the presence of an atmosphere.
Gas transition temperature is that at which atoms repel each other; every atom (or molecule) exists as an individual entity.
These conditions do not always occur at the same temperature and pressure.
Conclusion
It is now evident, if only from Fig 1, that the state of elemental matter - in all its forms and its properties# - may be calculated mathematically at any temperature and/or pressure simply from its atomic number, neutronic ratio and its lattice factor (ζ) (Fig 3).
# Tensile modulus, yield strength, tensile strength, elongation, shear modulus, bulk modulus, Poisson's ratio, expansivity, thermal conductivity, electrical, etc.
All that is required to verify this work is a comprehensive database of accurately defined elemental matter densities and gas transition temperatures established in a vacuum. But the facts are plain for all to see: the Newton-Coulomb atomic model has been further authenticated by applying it to the properties of matter.
CalQlata's consequential conclusions have been allocated to a dedicated webpage, reserving this page for the scientific facts alone.
Definition
The force (Fₘ) holding adjacent atoms together is the magnetic field generated by their proton-electron pairs. This force is constant because the magnetic charge (currently referred to as mass) responsible, is constant.
The force (Fₑ) repelling adjacent atoms is due to the positive electrical charge collected by their [nucleic] protons. This charge (e') varies linearly with the EME collected by the orbiting electrons from their surroundings and transferred to - and held by - their proton partners.
If the electrical force is less than the magnetic force, the matter will exist in a viscous state.
If the electrical force is greater than the magnetic force, the matter will exist in a gaseous state.
The gas transition temperature occurs when these two forces are equal (Fₘ = Fₑ).
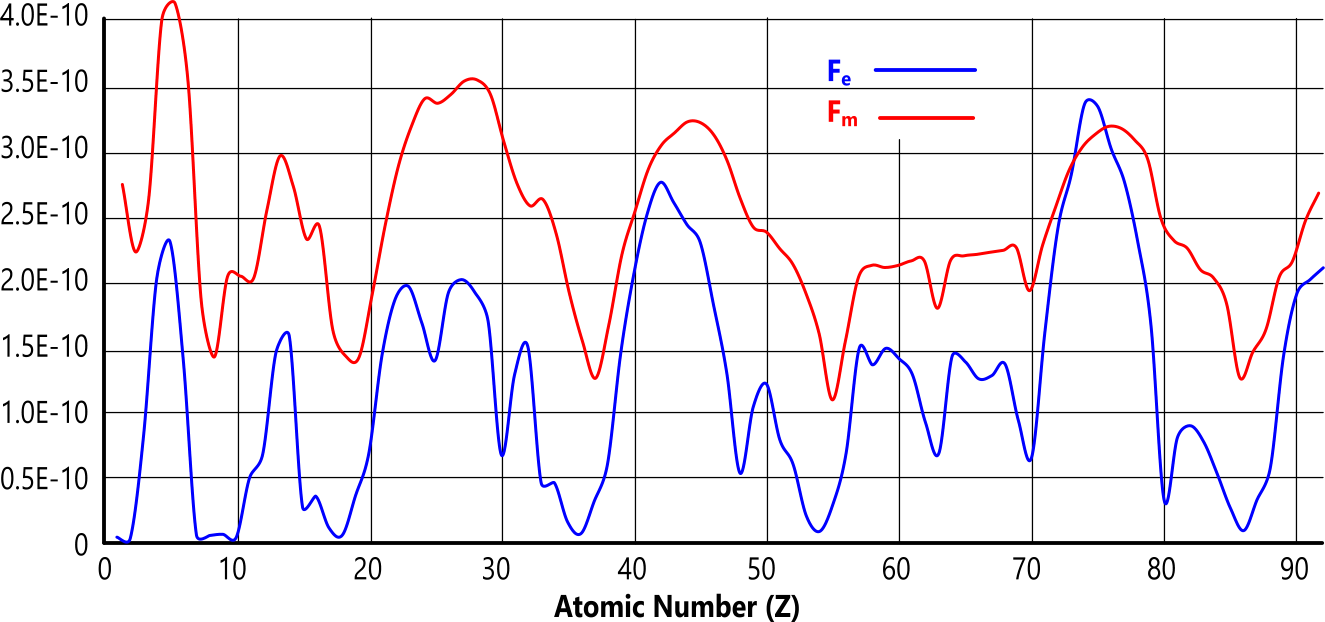
This relationship is the fundamental cause of the 'State of Matter' (Fig 2).
Gas-Transition Temperature
Gas transition temperature occurs when the electrical and magnetic forces are equal (Fₑ=Fₘ) (Fig 2)
The temperature at which this condition occurs here on Earth, depends upon the partial pressure of same-elemental atoms in the atmosphere.
Fig 1 reveals an undeniable relationship between the magnetic and electrical inter-atomic forces and the gas transition temperature of the elements.
Whilst documented values for the boiling-point of elemental matter are at best 'analogous', they do reveal a definite pattern (Fig 3). Their inconsistency is due to poorly controlled experimentation procedures along with the long-standing custom of copying data from unreliable sources.
However, the calculated values (CalQ), which are mathematically consistent and accurate, also follow the same pattern.
Gas-transition temperature occurs when both magnetic and electrical forces are equal; Fₘ = Fₑ:
kB.Ṯg/d = hₑ².mₚ/Y . (ζ.ψ/d)³
it can therefore be calculated like this:
Ṯg = hₑ².mₚ . (ζ. ψ)³ / kB.Y.d²
and Fig's 1 & 3 show conclusively that this calculation method is valid.
The gas-transition temperature of all matter declines with reducing ambient pressure and temperature.
And the gas-transition temperature (in a vacuum) is generally greater than the boiling-point of the same matter; in a vacuum or otherwise.
Viscous
The term 'viscosity' describes all and/or any matter when adjacent atoms are attracted to each other in solid or liquid form, and refers to the resistance in adjacent atoms to slip - plastically, as in plastic stress - relative to each other; Fₘ>Fₑ.
Liquid matter is no different to solid but the inter-atomic electrical separation force (Fₑ), which varies with temperature, is sufficient to reduce the effect of the attractive magnetic force (Fₘ) whereby slip between adjacent atoms occurs with little resistance (low viscosity). What we understand as 'solid' matter is simply that with a very high viscosity, you can still cause its atoms to slip by applying greater (than for liquid matter) force.
Liquidity refers to a body's ability to maintain its shape under the prevalent ambient conditions; gravitational acceleration, atmospheric pressure, etc. It will differ in all matter, for example, here on Earth and and on our moon.
Magnetism remains constant at any temperature (Fig 2), which is why the attraction force (Fₘ) between atoms is constant.
Gaseous
Gaseous matter describes adjacent atoms that repel each other; Fₘ<Fₑ.
This condition occurs when the electrical separation force (Fₑ) between adjacent atoms at elevated temperatures is sufficient to overcome (exceed) the attractive magnetic force (Fₘ).
This electrical force (Fₑ) is due to the positive electrical charge held in the nucleic protons, which varies with temperature (Fig 2).
As a gas, the force between adjacent atoms (F = Fₘ-Fₑ) is negative because Fₑ is greater than Fₘ, therefore, viscosity should not exist. However, at very low temperatures, just above gas transition, magnetic attraction (Fₘ) is still evident and responsible for the resistance in bodies travelling through an atmosphere.
Partial Pressure
At first sight, Dalton's law and partial pressure theory appear to conflict.
If as Dalton states; each gas in a mixture of gases should be treated independently, the pressure in a container of mixed gases should be the maximum individual pressure. But this is not the case; partial pressure theory tells us that the total pressure is the sum of the individual gases.
This anomaly may be explained as follows:
The positive electrical charge in all atomic protons (e') will repel all other atoms, irrespective of atomic number, but such repulsion is random.
All same-element atoms will repel each other equally according to their respective lattice structures, and because all energies (and forces) try to settle at their minimum condition, they will distribute evenly and equally throughout their container, maximising their relative distances (Dalton).
This is why; the total pressure in a container of mixed gases is the sum of all the individual pressures and same-elemental gases must be treated individually.
Dalton's Law
Dalton stated that each individual gas in a container of mixed gases, distributes independently.
It is also true that same-element atoms coalesce as a liquid.
These phenomena occur because an atom's treatment of its neighbouring atoms is defined by its nucleic arrangement.
e.g., if an elemental atom's nucleic protons are arranged in, say, a face-centre cubic pattern, this will be replicated in the arrangement of neighbouring same-element atoms in both gaseous and viscous condition.
In other words ...
In viscous state (below the gas transition temperature), each elemental atom will attract same-element atoms arranging themselves in the same pattern as its nucleic protons. I.e. the lattice structure of the viscous matter will also be face-centre cubic.
In gaseous state (above the gas transition temperature), each elemental atom will repel same-element atoms according to the same pattern as its nucleic protons. I.e. same-element gaseous atoms in a container will be organised [repulsed] in a face-centre cubic pattern, causing all same-element atoms to fill the container independently.
It is their unique gas transition temperatures and nucleic structures that ensure same-element atoms coalesce (as a liquid) and repel evenly (as a gas).
Partial pressure theory states that the total pressure inside a container of mixed gases is the sum of all individual same-element [partial] gas pressures.
It is also true that the internal [core] pressure of a solid comprising numerous elemental atoms, is the sum of their individual core pressures.
Irrespective of its nucleic structure, an elemental atom will always attract or repel neighbouring atoms according to their respective gas transition temperatures and nucleic arrangements.
In other words ...
Dalton's Law applies to all elements in both gaseous and viscous conditions.
Lattice Structure
Same element atoms always attract and repulse in a similar pattern to that held by the protons in an atom's nucleus. This pattern is what we today refer to as a lattice structure that depends upon the atom's neutronic ratio.
We designate these lattice structures as; face-centre cubic, body-centre cubic, tetrahedra, close-packed hexagonal, etc. but they are not quite so simple. Whilst a particular group of atoms may resemble a single structure, they will not be identical; every lattice structure differs from every other.
This pattern applies to all same-element matter in both viscous and gaseous form, and is therefore responsible for partial pressure (gas) theory.
Inter-Atomic Spacing
An average value for atomic spacing (d), which is due to inter-atomic forces, may be found from its density and atomic mass; d = ³√[mₐ/ρ].
Whilst 'd' will vary with lattice structure and orientation in a crystal of viscous elemental matter, it is not so in amorphous matter, where 'd' will be similar in any direction.
However, whilst 'd' tends to be constant, irrespective of temperature - due to magnetism - minor growth will occur at elevated temperatures due to a significant reduction in δF (Fₘ-Fₑ), and lattice structure variation..
Inter-Atomic Forces
Every proton-electron pair in every atom generates a magnetic field force that holds adjacent atoms together, and a variable positive electrical charge (eꞌ), which forces them apart. The highest of these forces are generated by the proton-electron pairs in shell-1. PE₁ is therefore the dominant potential energy.
Given that we know that PVRT is a well-established and recognised method of calculating the pressure of a gas, we can use it as a means of verification for the calculation of inter-atomic forces:
p = Rᵢ . Ṯ₁.ρ / N.(RAM/1000) {N/m²}
But we can also calculate it like this ...
p = -PE₁ / Y.d³ = kB.Ṯ₁ / d³ {N/m²}
… because all three methods generate exactly the same result.
As force is pressure multiplied by area, the electrical repulsion force between adjacent atoms may be calculated thus:
Fₑ = p.d²
Fₑ = PE₁ / Y.d = kB.Ṯ₁ / d
Fₑ = μ.I₁² . (2π)² . R₁/d / Y
Fₑ = k.e' / (d.Rₙ.FT)
all of which give exactly the same result,
… and inter-atomic attraction force may be calculated thus:
Fₘ = hₑ².mₚ/Y . (ψ.ζ/d)³ {N}
It is interesting to note that the magnetic field generated by an atom's outermost proton-electron pair(s) gives exactly the same result. In other words:
Fₘ = μₒ . Iₛ² . (2π)² . (ψ.Rₛ/d)³ . ξₘ/Y = hₑ².mₚ/Y . (ψ/d)³
Where (subscript-1 refers to shell-1 value):
N = number of atoms in molecule (1 or 2)
ρ = matter density (gaseous and viscous)
d = inter-atomic spacing (³√[mₐ/ρ])
mₐ = atomic mass
ψ = neutronic ratio
Ṯ₁ = measured temperature of the matter
PE₁ = potential energy
R = electron's orbital radius
I = orbiting electron's current (e.ƒ)
ƒ = electron's orbital frequency
sub-script; '₁' refers to an atom's innermost shell (shell-1)
sub-script; 'ₛ' refers to an atom's outermost shell
FT = temperature factor:
ϵ₁ = 0.0226720208177874
ϵ₂ = 6918047.99908078
ϵ₃ = (ϵ₂-ϵ₁)/(Ṯₙ-Ṯₓ)
ϵ₄ = 4.63629997675112
(ϵ - ϵ₁)/(Ṯ₁ - Ṯₓ) = (ϵ₂ - ϵ₁)/(Ṯₙ - Ṯₓ)
ϵ = (Ṯ₁ - Ṯₓ).(ϵ₂ - ϵ₁)/(Ṯₙ - Ṯₓ) + ϵ₁
ϵ₃ = (ϵ₂ - ϵ₁)/(Ṯₙ - Ṯₓ)
ϵ = (Ṯ₁ - Ṯₓ).ϵ₃+ ϵ₁
ϵ = ϵ₄ . [(Ṯ₁ - Ṯₓ).ϵ₃+ ϵ₁]
FT = Rₙ.ϵ
Ṯ₁ = measured temperature of atom (shell-1 temperature)
Oxygen is the control element; @ 90.1 K FT/ϵ₄ = 1
Both of these forces (Fₘ & Fₑ) are equal at gas transition temperature.
Viscous matter: Fₘ > Fₑ
Gas transition: Fₘ = Fₑ
Gaseous matter: Fₘ < Fₑ
It is evident from Figs 5a & 5b that the inter-atomic force calculation method is correct;
Fig 5a shows the correct elements in gaseous state at atmospheric temperature here on earth, and
Fig 5b shows the only two elements that have a higher gas-transition temperature than that at the surface of our sun; tungsten, rhenium & osmium (5755K) being very close.
Finally, the internal pressure generated within any matter may be derived using the above inter-atomic forces thus:
p⁺ᵛᵉ = kB.Ṯ₁ / d³
p⁻ᵛᵉ = hₑ².mₚ.(ψ.ζ)³ / Y.d⁵
δp = p⁺ᵛᵉ - p⁻ᵛᵉ
If δp is greater than zero at temperature Ṯ₁, the matter will exist as a gas.
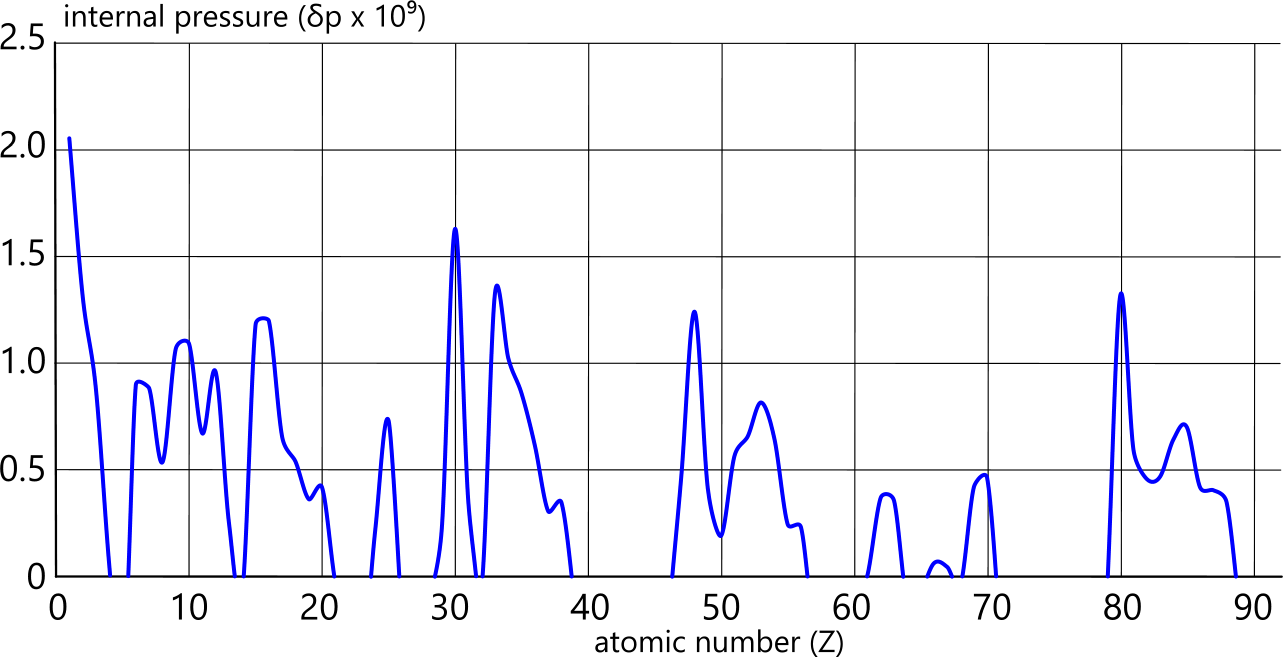
Figure 5c shows which elements exist in a gaseous state at 3000K
Important Note: The ‘PVRT’ repulsion pressure formula applies equally to viscous and gaseous matter.
Specific Heat Capacity (SHC)
The specific heat capacity for all atoms, may be calculated thus; SHC = ΣKE / Ṯ.Y.mₐ
where ΣKE is the sum of the kinetic energies in all the proton-electron pairs in any atom, and mₐ is the atomic mass (Fig 6).
Fig 6 confirms the Newton-Coulomb atomic model with two electrons per orbital shell.
Properties
These forces (Fₘ & Fₑ) can now be used to predict various properties of matter, which when taken along with the relationship between neutronic ratio (ψ) and matter density (ρ) (Fig 3), it is evident that all the physical properties of matter can be predicted from an atom's neutronic ratio and its atomic mass. For example:
F = Fₘ-Fₑ
However, we now know that the properties of viscous elemental matter may also be calculated using the PVRT formulas:
Viscosity
The dynamic viscosity (μ) of all elements - at a specified temperature - can be calculated thus;
μ = p / Γ.ƒ
Note: the kinematic viscosity (ν) may be derived thus; ν = μ/ρ
Surface Tension
Surface tension is the linear force holding adjacent atoms together. It is measured in units of N/m and can be calculated thus;
γ = p.Y.d
Elastic Modulus
Young's modulus (E) for each element at a specified temperature may be determined thus;
E = p . (d/(h.sin(60°))³
where h = d.(1-sin(60°))
The unmistakeable relationship between documented values at room-temperature and those calculated here may be seen in Fig 7
Yield Stress
The yield stress of elemental viscous matter is simply a measurement of the internal pressure between atomic planes;
σᵧ = p
Noble gases
Fig 8 reveals the noble gases based upon the neutronic ratio, which may be calculated thus;
Γ = 9.(ψ -1)
the noble gases occur when Γ is at or close to an integer; 1 to 5
We can also calculate ...
Radial separation: Rₛ = ³√[mₐ/ρ]
Gas pressure: p = Fₑ/Rₛ²
Tensile stress: σ = ν.F/Rₛ²
Yield stress: σᵧ = Fₑ/d² . Fₛ
where the shear factor (Fₛ) may be calculated thus: Fₛ = d/h
Atmospheric drag: Fd = hₑ.ρ.ψ . N° . (vd/vₐ)² . (PEₙ/-PE₁)
etc.
... using the same inter-atomic forces.
In fact, it is highly likely that viscosity and gas pressure are the only genuine properties we need to consider when describing the behaviour of elemental matter given their dependence on temperature; along with the fact that viscous and gaseous are its only possible states.
Atmospheric Drag
The current method for calculating the resistance (force) generated in a body travelling through a gas was established by J. R. Morrison et. al.
However, because the magnetic [attraction] force (Fₘ) between adjacent atoms is responsible for the drag on bodies passing through an atmosphere, the Newton-Coulomb atomic model can now be used to determine this resistance based upon relative velocities and energies thus:
F = hₑ.ρ.ψ . N° . (vd/vₐ)² . (PEₙ/-PE₁)
where;
A = cross-sectional area
ρ = gas (e.g. air) density
N° = number of atoms in contact with the body (= A / π.Rₛ²)
vd = relative velocity between body and gas
v₁ = electron velocity in shell-1 (gas-atom)
PE₁ = potential energy proton-electron pair in shell-1 (gas-atom)
Whether or not inter-atomic magnetism is the cause of atmospheric drag, the above calculation makes this argument extremely compelling; yet again validating this atomic model.
The Molecule
The molecule (and atomic bonding) is the next step, which can also be solved mathematically using the same atomic forces.
Whilst Keith Dixon-Roche knows how to solve this problem,
due to the responses he has received as a result of his scientific discoveries and their possibilities, he has decided to leave this work up to you,
but he is willing to give you a hint:
'atomic and matter densities react differently with varying temperature;
e.g. the density of an iron atom @ 300K is 0.007533336 kg/m³ but the density of its matter is 7870 kg/m³.
That atomic density increases with rising temperature as matter density decreases, is a clue as to the functioning of chemical bonding; electron charge sharing.'.
Oh yes! and there is no such thing as co-valent boding. Such bonding between atoms is due to quite a different function.
He wishes you luck.
Notes
- Refer to our elemental data web-page..
Note: the value included in Elements was taken from the most reliable documented source at the time. However, whilst CalQlata's calculated value may not be exact, it clearly demonstrates that none of the documented values can be counted upon. - The density of solid boron is said to be 2320 kg/m³, but a reliable technical source (page 5) states that the specific gravity of liquid boron varies between 1.32 and 1.36 but does not state the associated temperatures or pressures.
- H⁺, which represents about 99.997% of all atmospheric hydrogen, can never coalesce as a liquid because it possesses a positive charge and generates no magnetic field. However, the inability of hydrogen atoms (H) to attract other hydrogen atoms (H) in viscous form is currently hypothesis, based upon their positive proton electrical charges. If, however, at a sufficiently low temperature, their attractive magnetic forces (Fₘ) are greater than their repulsive electrical forces (Fₑ), a liquid state may exist.
Further Reading
You will find further reading on this subject in reference publications(69, 70, 71 & 73)